On the Cauchy problem for nonlinear evolution equations and regularity of solutions
Abstract
In some previous works a generalized implicit function theorem of Nash-Moser type has been applied to prove the local well posedness for the Cauchy problem for several types of nonlinear evolution equations. For istance, applications of this new method have been given to ordinary differential equations in Fréchet spaces,to nonlinear parabolic partial differential equations and to some specific nonlinear Schrödinger type equations. All these results take ![C<sup>1</sup>([a,b],E)](http://212.189.136.205/plugins/generic/latexRender/cache/643f2bf9fe3898e8ba129436359fcfd0.png) as the basic function space where E is a general Fréchet space or
as the basic function space where E is a general Fréchet space or 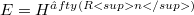 , respectively. The purpose of this note is to show that a similar approach based on Nash-Moser techniques works with the function space
, respectively. The purpose of this note is to show that a similar approach based on Nash-Moser techniques works with the function space ![C^∈fty([a,b],E)](http://212.189.136.205/plugins/generic/latexRender/cache/8133a5b41ff285bbd70ea0dbf61dc8d3.png) as well providing the existence of
as well providing the existence of 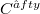 -solutions smoothhly depending on the initial value. In particular, sufficient conditions on E are given suche that
-solutions smoothhly depending on the initial value. In particular, sufficient conditions on E are given suche that ![C^∈fty ([a,b],E)](http://212.189.136.205/plugins/generic/latexRender/cache/202e742b6b4a4cbd8276c48fc0cff18a.png) satisfies a required smoothing property.
satisfies a required smoothing property.
![C<sup>1</sup>([a,b],E)](http://212.189.136.205/plugins/generic/latexRender/cache/643f2bf9fe3898e8ba129436359fcfd0.png) as the basic function space where E is a general Fréchet space or
as the basic function space where E is a general Fréchet space or 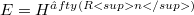 , respectively. The purpose of this note is to show that a similar approach based on Nash-Moser techniques works with the function space
, respectively. The purpose of this note is to show that a similar approach based on Nash-Moser techniques works with the function space ![C^∈fty([a,b],E)](http://212.189.136.205/plugins/generic/latexRender/cache/8133a5b41ff285bbd70ea0dbf61dc8d3.png) as well providing the existence of
as well providing the existence of 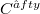 -solutions smoothhly depending on the initial value. In particular, sufficient conditions on E are given suche that
-solutions smoothhly depending on the initial value. In particular, sufficient conditions on E are given suche that ![C^∈fty ([a,b],E)](http://212.189.136.205/plugins/generic/latexRender/cache/202e742b6b4a4cbd8276c48fc0cff18a.png) satisfies a required smoothing property.
satisfies a required smoothing property.DOI Code:
10.1285/i15900932v17p13
Keywords:
Cauchy problem; Nonlinear evolution equation; Well posedness; Nash-Moser technique; Implicit function theorem; Smoothing property; Ordinary differential equation; Fréchet space; Nonlinear parabolic equation; Nonlinear Schrödinger equation
Classification:
35F25; 58C15; 34G20; 35Q55
Full Text: PDF


