Parameter functional dependence in an electrochemical model: theoretical and computational issues
Abstract
In this article we study the simplest parameter dependent ODE model that allows to describe the electrochemical impedance as a curve(Error rendering LaTeX formula) in the complex plane. The parameters of the original ODE having a straightforward physical meaning appear in 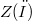 combined in a highly nonlinear form. Usually, a nonlinear least squares procedure is applied to identify these parameters by fitting experimental impedance data and, as shown in [2], this can yield an ill-posed and ill-conditioned problem. In fact, several sets of different parameters,called Numerical Global Minima (NGM) can be identified that produce undistinguishable fitting curves.In this paper, we show that: 1) ill- posedness can be avoided by working in a different parameter space, where the new parameters have a physical meaning that is different from the traditional one but nevertheless exhibit a clear relationship with them, and a unique optimal set can be identified; 2) there exist curves of NGMs in the original space.
combined in a highly nonlinear form. Usually, a nonlinear least squares procedure is applied to identify these parameters by fitting experimental impedance data and, as shown in [2], this can yield an ill-posed and ill-conditioned problem. In fact, several sets of different parameters,called Numerical Global Minima (NGM) can be identified that produce undistinguishable fitting curves.In this paper, we show that: 1) ill- posedness can be avoided by working in a different parameter space, where the new parameters have a physical meaning that is different from the traditional one but nevertheless exhibit a clear relationship with them, and a unique optimal set can be identified; 2) there exist curves of NGMs in the original space.
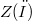 combined in a highly nonlinear form. Usually, a nonlinear least squares procedure is applied to identify these parameters by fitting experimental impedance data and, as shown in [2], this can yield an ill-posed and ill-conditioned problem. In fact, several sets of different parameters,called Numerical Global Minima (NGM) can be identified that produce undistinguishable fitting curves.In this paper, we show that: 1) ill- posedness can be avoided by working in a different parameter space, where the new parameters have a physical meaning that is different from the traditional one but nevertheless exhibit a clear relationship with them, and a unique optimal set can be identified; 2) there exist curves of NGMs in the original space.
combined in a highly nonlinear form. Usually, a nonlinear least squares procedure is applied to identify these parameters by fitting experimental impedance data and, as shown in [2], this can yield an ill-posed and ill-conditioned problem. In fact, several sets of different parameters,called Numerical Global Minima (NGM) can be identified that produce undistinguishable fitting curves.In this paper, we show that: 1) ill- posedness can be avoided by working in a different parameter space, where the new parameters have a physical meaning that is different from the traditional one but nevertheless exhibit a clear relationship with them, and a unique optimal set can be identified; 2) there exist curves of NGMs in the original space.DOI Code:
10.1285/i15900932v27n1p39
Keywords:
Ordinary differential equation; Nonlinear least squares; Electrochemical impedance; Parameter identification
Full Text: PDF PS


